K Traveling Repairman Problem

The resulting problem called weighted k-traveling repairman problem wkTRP is a generalization of the well-known traveling repairman problem and can be used to model a variety of real-world. To solve the wkTRP we propose an iterated local search heuristic and an exact branch-and-cut algorithm enriched with valid inequalities.

Hybrid Evolutionary Search For The Traveling Repairman Problem With Profits Sciencedirect

The resulting problem called weighted k-traveling repairman problem wkTRP is a generalization of the well-known traveling repairman problem and can be used to model a variety of real-world applications.

K traveling repairman problem. The goal is to find tours on which to send the repairmen that minimize the average time a customer has to wait. The stochastic profitable k-traveling repairman problem aimed at finding a subset of demand nodes to be served and the order of visiting them by a fleet of k vehicles such that the total revenue is maximized. The waiting time of a customer v at position i in given as input a metric d on a set of vertices V and the optimal tour M LT1 is lower-bounded by the a special vertex s V which will be the starting cost of the i-MST solution.

Its easy to see that pointdepot. We consider the k-traveling repairman problem a generalization of the metric traveling repairman problem also known as the minimum latency problem to multiple repairmen. In these problems waiting time or latency of a customer is defined as the time passed from the beginning of the travel until this customers service completed.

We consider the k-traveling repairman problem a generalization of the metric traveling repairman problem also known as the minimum latency problem to multiple repairmen. The resulting problem called weighted k-traveling repairman problem wkTRP is a generalization of the well-known traveling repairman problem and can be used to model a variety of real-world applications. We give an 8497-approximation algorithm for this generalization where denotes the best achievable approximation factor for the problem of nding the least cost rooted tree spanning i vertices i-MST problem.

This problem is a generalization of the metric traveling repairman problem TRP which is also known as the deliveryman problem and the minimum latency problem. The multiple traveling repairman problem kTRP is a generalization of the traveling repairman problem which is also known as the minimum latency problem and the deliveryman problem. Considering the complexity of the problem inherited from the incorporation of the.

We give a polynomial-time 8497α-approximation algorithm for this generalization where α denotes the best achievable approximation factor for the problem of finding the least-cost rooted tree spanning i vertices of a metric. Informally in the k-traveling repairman problem we are given that there are k repairmen at a common depot s and n customers sitting in some metric space at prescribed distances from each other and the depot. We give a polynomial-time 8497 -approximation algorithm for this generalization where denotes the best achievable approximation factor for the problem of nding the least-cost rooted tree spanning i vertices of a metric.

We give an 8497-approximation algorithm for this generalization where denotes the best achievable approximation factor for the problem of nding the least cost rooted tree spanning i vertices i-MST problem. The set-up cost associated with each job depends not only on the job that precedes it but also on its position time in the sequence. We consider the k-traveling repairmen problem also known as the minimum latency problem to multiple repairmen.

We consider the k-traveling repairmen problem also known as the minimum latency problem to multiple repairmen. Given an undirected graph GVE and a source vertex s Vthek-traveling repairman KTR problem also known as the minimum latency problem asks for k tours each starting at s and together covering all the vertices customers such that the sum of the latencies experienced by the customers is minimum. The time-dependent traveling salesman problem may be stated as a scheduling problem in which n jobs have to be processed at minimum cost on a single machine.

For the latter problem a 2 ε-approximation is known. Informally in the k-traveling repairman problem they are given that there are k repairmen at a common depot s and n customers sitting in some metric space at prescribed distances from each other. Given a finite metric on a set of vertices V and a source vertex s V the k-traveling repairman KTR problem a generalization of the metric traveling repairman problem also known as the minimum latency problem the delivery man problem and the school bus-driver problem asks for k tours each starting at s depot and together covering all the vertices customers such that the sum of the latencies experienced by the customers is minimum.

The resulting problem called weighted k-traveling repairman problem wkTRP is a generalization of the well-known traveling repairman problem and can be used to model a variety of real-world. This paper deals with an optimization problem encountered in the field of transport of goods and services namely the K-traveling repairman problem K-TRP. In the k-traveling repairman problem we are 1.

Given an undirected graph GVE and a source vertex s Vthek-traveling repairman KTR problem also known as the minimum latency problem asks for k tours each starting at s and together covering all the vertices customers such that the sum of the latencies experienced by the customers is minimum. This project is a utility that makes repairs to the Windows 7 or 81 Task schedule. We consider the k-traveling repairman problem a generalization of the metric traveling repairman problem also known as the minimum latency problem to multiple repairmen.

The optimization method described here combines finding. In particular it fixes problems where opening the Task Scheduler or trying to configure Windows Backup results in the message The task image is corrupt or has been tampered with 0x80041321. Traveling repairman problem also known as the minimum latency problem to multiple repairmen.

We give an 8497α-approximation algorithm for this generalization where α denotes the best achievable approximation factor for the problem of finding the least cost rooted tree spanning i vertices i -MST problem.

Memetic Algorithm For The Multiple Traveling Repairman Problem With Profits Sciencedirect

Combining Traveling Salesman And Traveling Repairman Problems A Multi Objective Approach Based On Multiple Scenarios Sciencedirect

Kecotabs Professional Paintless Dent Repair Products Metal Fabrication Tools Auto Body Repair Moving Tools

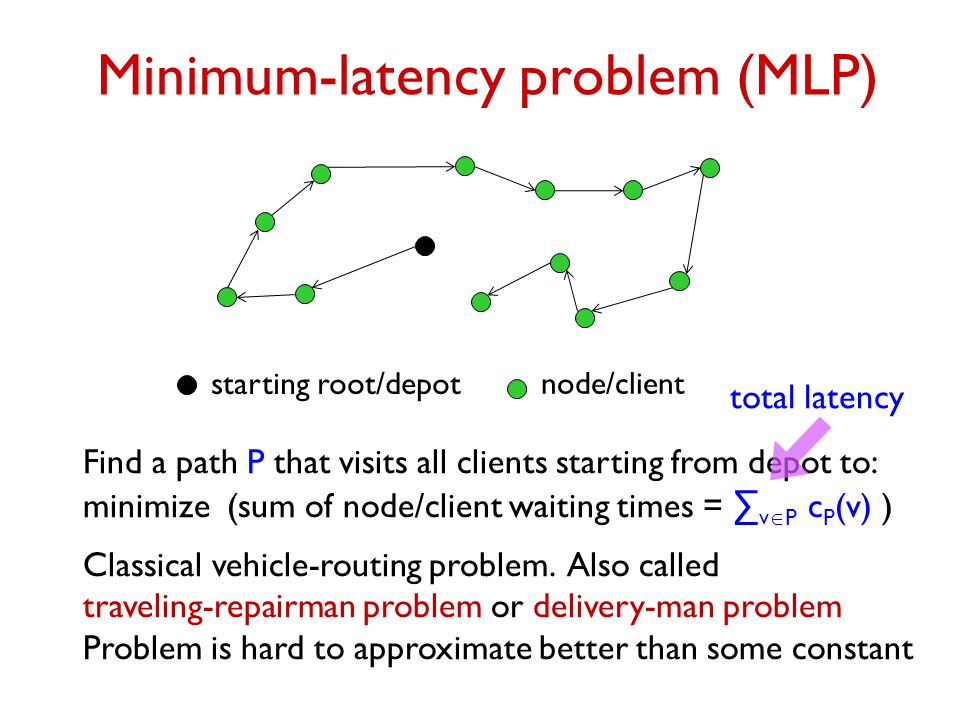Lp Based Approximation Algorithms For Multi Vehicle Minimum Latency Problems Chaitanya Swamy University Of Waterloo Joint Work With Ian Post University Ppt Download

If You Still Frustrated To Think About Your Electricitybill And You Want To Know T Heating And Air Conditioning Air Conditioner Repair Air Conditioning Repair

Traveling Salesman Problem Using Branch And Bound Geeksforgeeks

How To Be A Traveling Esthetician Esthetician Esthetics Esthetician School

Important Points To Consider Concerning The Roof On Your Home In 2020 Roofing Roof Best Location

A Grasp With Iterated Local Search For The Traveling Repairman Problem With Profits Sciencedirect

Https Ieeexplore Ieee Org Iel7 7502491 7510595 07511247 Pdf

Handyman Or Repairman Business Management Excel Spreadsheet To Track All Jobs Income Expenses And Handyman Business Business Management Pricing Calculator